Page 30 - eEISBN FINALIZE

= cos

= 150 kos 30

= 129.9

2.5 THE RESULTANT FORCE OF COPLANAR FORCES BY ADDITION

When a system of more than two forces have obtained, it is much more convenient and easier to

find the components each force along the specified axes. Add these components algebraically and

then form the resultant. This way proved to be easier than forming the resultant of the forces by
successive application of the parallelogram law as discussed before.

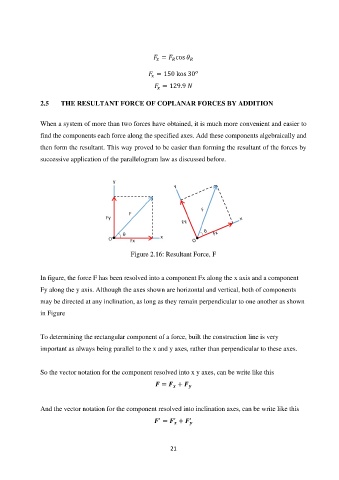
Figure 2.16: Resultant Force, F

In figure, the force F has been resolved into a component Fx along the x axis and a component

Fy along the y axis. Although the axes shown are horizontal and vertical, both of components
may be directed at any inclination, as long as they remain perpendicular to one another as shown

in Figure

To determining the rectangular component of a force, built the construction line is very

important as always being parallel to the x and y axes, rather than perpendicular to these axes.

So the vector notation for the component resolved into x y axes, can be write like this
= +

And the vector notation for the component resolved into inclination axes, can be write like this
′
′
′
= +

21