Page 42 - EBOOK RISK MANAGEMENT

Dependent outcomes is a situation when the occurence of outcome A will highly

affect the outcome B. The probability that dependent events A and B occur
together is P(A and B) = P(A) × P(B given A) where P(B given A) means the probability

of event B given the occurrence of event A. This principle can be extended to any

number of individual events. As an example, house A is only two feet away from
house B. If house A caught fire, probability of house B will be burned is very high. If

there are two separate outcomes such as house A and house B caught fire, the
probability of both outcomes will occur is determined by multiplysing the probability

of A by the probability of B, given that A has occured.
If: P (A) = 0.03

P (B|A) = 0.80

Therefore: P(A and B) = P (A) x P (B|A)
= 0.03 x 0.80

= 0.024

4.3.3 Expected Value

The expected value is also known as the expectation, mathematical expectation,
EV, average, mean value, mean, or first moment. The expected value can be used

to calculate loss frequency and loss severity which is denoted as E[X]. There are

two ways to calculate the expected value either using probability () or frequency
() of distribution.

∑
[] = ∑ [] =
∑

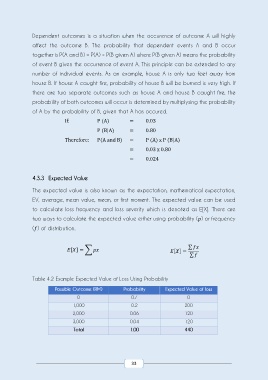
Table 4.2 Example Expected Value of Loss Using Probability

Possible Outcome (RM) Probability Expected Value of loss

0 0.7 0
1,000 0.2 200
2,000 0.06 120
3,000 0.04 120
Total 1.00 440

33