Page 11 - DJJ20063- Thermodynamics 1

DJJ20063- Thermodynamics 1

All other physical quantities, which can be expressed in terms of one or more of these,

are known as ‘derived quantities’. Physical quantities like area, volume, density, velocity,

acceleration, force, energy, power, torque etc. are called derived quantities since they

depend on one or more of these fundamental quantities. The units of the derived
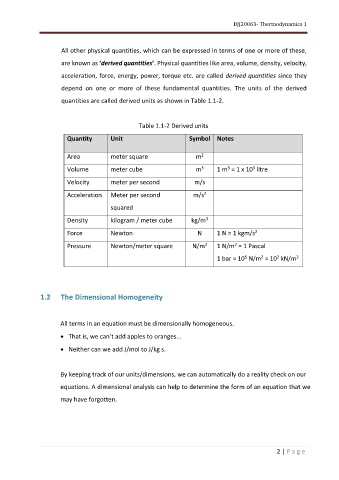
quantities are called derived units as shown in Table 1.1-2.

Table 1.1-2 Derived units

Quantity Unit Symbol Notes

2
Area meter square m
3
3
3
Volume meter cube m 1 m = 1 x 10 litre
Velocity meter per second m/s
Acceleration Meter per second m/s 2

squared

Density kilogram / meter cube kg/m 3
Force Newton N 1 N = 1 kgm/s 2

2
2
Pressure Newton/meter square N/m 1 N/m = 1 Pascal
2
5
2
2
1 bar = 10 N/m = 10 kN/m

1.2 The Dimensional Homogeneity

All terms in an equation must be dimensionally homogeneous.

• That is, we can’t add apples to oranges…

• Neither can we add J/mol to J/kg s.

By keeping track of our units/dimensions, we can automatically do a reality check on our

equations. A dimensional analysis can help to determine the form of an equation that we

may have forgotten.

2 | P a g e