Page 112 - ebook fluid mechanics_finalize

FLUID MECHANICS

f. Simple Pitot Tube

h

H
A B

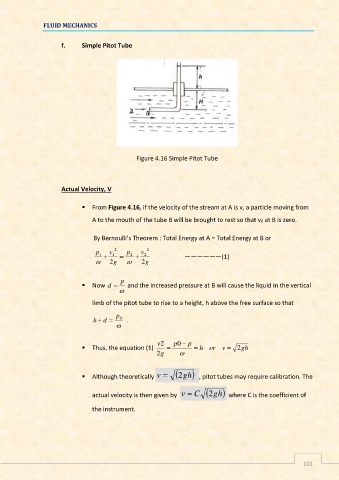
Figure 4.16 Simple Pitot Tube

Actual Velocity, V

▪ From Figure 4.16, if the velocity of the stream at A is v, a particle moving from

A to the mouth of the tube B will be brought to rest so that v0 at B is zero.

By Bernoulli’s Theorem : Total Energy at A = Total Energy at B or
p 1 + v 1 2 = p 2 + v 2 2
 2 g  2 g ——————(1)

p
▪ Now d = and the increased pressure at B will cause the liquid in the vertical


limb of the pitot tube to rise to a height, h above the free surface so that
p
h + d = 0 .


v2 p0 − p
▪ Thus, the equation (1) = = h or v = 2 gh
2 g 

▪ Although theoretically v = ( gh2 ) , pitot tubes may require calibration. The

actual velocity is then given by v = C ( gh2 ) where C is the coefficient of

the instrument.

101