Page 101 - DJJ20063- Thermodynamics 1

DJJ20063- Thermodynamics 1

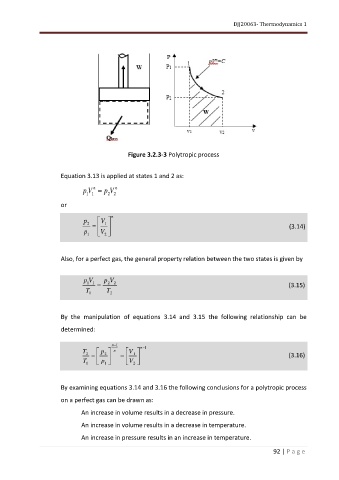
Figure 3.2.3-3 Polytropic process

Equation 3.13 is applied at states 1 and 2 as:

n
n
p 1 V = p 2 V
2
1
or
p  V  n
2 =  1  (3.14)
V
p 1  2 

Also, for a perfect gas, the general property relation between the two states is given by

p V p V
1 1 = 2 2 (3.15)
T 1 T 2

By the manipulation of equations 3.14 and 3.15 the following relationship can be

determined:

n − 1 n − 1
T  p  n V 
2 =  2  =  1  (3.16)
T 1  p 1   V 2 

By examining equations 3.14 and 3.16 the following conclusions for a polytropic process

on a perfect gas can be drawn as:

An increase in volume results in a decrease in pressure.
An increase in volume results in a decrease in temperature.

An increase in pressure results in an increase in temperature.

92 | P a g e