Page 91 - DJJ20063- Thermodynamics 1

DJJ20063- Thermodynamics 1

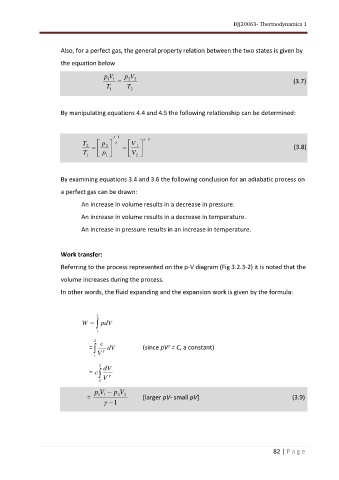
Also, for a perfect gas, the general property relation between the two states is given by

the equation below

p V p V
1 1 = 2 2 (3.7)
T 1 T 2

By manipulating equations 4.4 and 4.5 the following relationship can be determined:

 − 1  − 1
T  p   V 
2 =  2  =  1  (3.8)
T 1  p 1   V 2 

By examining equations 3.4 and 3.6 the following conclusion for an adiabatic process on
a perfect gas can be drawn:

An increase in volume results in a decrease in pressure.

An increase in volume results in a decrease in temperature.

An increase in pressure results in an increase in temperature.

Work transfer:

Referring to the process represented on the p-V diagram (Fig 3.2.3-2) it is noted that the

volume increases during the process.
In other words, the fluid expanding and the expansion work is given by the formula:

2
W =  pdV
1
2 c

=   dV (since pV = C, a constant)
1 V
2 dV
= c  
1 V
p V − p V
= 1 1 2 2 [larger pV- small pV] (3.9)
 − 1

82 | P a g e